Enhanced: Simple Rules with Complex Dynamics

Sir Robert May [HN19] ^*

In the 19th century and the early years of the 20th century, deaths from infectious diseases fell dramatically in developed countries, well before the advent of antibiotics or vaccination [HN1]. The pattern illustrated for measles [HN2] (see the figure) can be repeated for scarlet fever, diphtheria, and other infections that carried off heroines in Victorian novels. The causes of the decline remain debatable, although a mixture of better hygiene, improved nutrition, and possible genetic changes in both human and viral or bacterial populations seems plausible (1, 2).

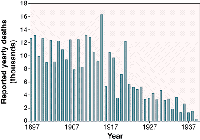
Understanding measles epidemics. Yearly numbers of deaths attributed to measles in England and Wales, 1897 to 1939 [from (1)].

This decline prompted the U.S. Surgeon General to declare in 1967 that "the time has come to close the book on infectious diseases." However, this statement is not true in the developing world, where measles, for example, kills millions of children each year (albeit aided by malnutrition) [HN3]. And the advent of HIV/AIDS [HN4] throughout the world, and of BSE ("mad cow disease") [HN5] in some nonhuman animal populations in developed countries, along with strains of tuberculosis [HN6] and other potentially lethal infections that are resistant to most antibiotics [HN7], underlines the continuing need for better understanding of the transmission and control of infectious diseases. To this end, Earn and colleagues [HN8] (3) report on page 667 of this issue a simple mathematical model that explains the cycles of childhood measles epidemics and how they fluctuate in response to the introduction of vaccination and variations in birthrate.

The past 20 years or so have seen many advances in the use of mathematical models to study the dynamics of the engagement between populations of human hosts and infectious pathogens. Such models for viral and bacterial infections commonly divide the host population into categories or compartments--those who have not yet experienced infection ("susceptibles," S); infected but not yet infectious ("exposed," E); infectious (I); recovered and thereby immune (R)--with various rate processes determining the flows into and out of each category. Recent work on these so-called "microparasitic" [HN9] models (1) builds on pioneering earlier studies in mathematical epidemiology in two principal ways. First (and often using newly available techniques), there is much more emphasis on assessing the models' parameters based on empirical data such as the transmission rates of a disease [HN10]. An important complication here is that transmission rates will often vary over the year, depending on the opening and closing patterns of schools. Second, relatively recent work on chaos in systems exhibiting nonlinear dynamics [HN11] has demonstrated how very simple sets of deterministic equations, such as those of the SEIR (Susceptible-Exposed-Infectious-Recovered) model [HN12], can explain exceedingly complex behavior.

Earn et al. (3) give a particularly revealing account of this mathematical frontier for measles incidence in four representative large cities (London, Liverpool, New York, and Baltimore) over a 30-year period that spans the introduction of vaccination. Before mass vaccination programs began in the 1960s, measles outbreaks showed different patterns in different places: annual cycles in many developing countries such as Kenya; roughly 2-year cycles in larger developed countries such as Denmark or the United Kingdom; and irregular outbreaks in small countries like Iceland. Under vaccination, patterns of measles outbreaks [HN13] have tended to become more irregular, both in time and location. Earn and co-workers show that the relatively simple SEIR model can synthesize many of these epidemiological observations, and at the same time can provide population biologists with real-world examples of chaos and bifurcation in the patterns of infectious disease incidence.

A central ecological concept for any population is the "basic reproductive ratio," R₀ [HN14]. In essence, this measures the average number of offspring an individual is capable of producing. For a microparasitic infection, R₀ is more precisely defined as the average number of secondary infections produced by one infected individual in a population where everyone is susceptible. Biologically, R₀ can be written as <b>T, where <b>is the average rate of producing infections per unit time, and T is the duration of infection. Empirically, for an endemic infection (such as measles before vaccination) R₀ can be estimated as the reciprocal of the fraction of the population who are susceptible, which can be estimated from serological surveys. For example, R₀ in a typical developed country before vaccination is around 5 to 10 for rubella, and around 20 for measles (1).

For the standard SEIR model, earlier studies (4) had emphasized that vaccinating a proportion, p, of the population had the effect of reducing <b>to <b>(1 - p). Correspondingly, R₀ is reduced by a factor of (1 - p). The criterion for ultimate eradication of infection is to drive the effective value of the basic reproductive ratio below unity: hence the eradication criterion p > 1 - 1/R₀. Earn et al. make generalizations from this observation, noting that changes in the birthrate similarly can be incorporated as effective changes in <b>. This means that, for a discussion of the dynamics of this SEIR system, all changes in either vaccination rates or birthrates can be incorporated in a single parameter--the effective value of the average transmission rate, <b>.

On the other hand, the dynamics of the system depend on the details--the amplitude and the shape--of the annual variation in the measles transmission rate. The system is essentially a forced pendulum, with the pendulum's inherent period dependent on <b> (which embraces any effects of changing birthrates or vaccination rates) and with a complicated forcing function (set by the seasonal details of school schedules). Earn et al. can thus summarize the dynamics, for any specified seasonal forcing pattern, in a single bifurcation diagram [HN15], which depends only on the effective value of the average transmission rate, <b> (Fig. 1 in their report) (3). In this way, they can then lay bare the otherwise bewilderingly different patterns of measles incidence seen (after the advent of vaccination) in London, Liverpool, New York, and Baltimore (3).

I think this does not, however, close the book on the topic. Earn et al. use the standard SEIR equations, with their "mass action" assumption that new cases arise in simple proportion to the product of the number of individuals who are susceptible and the number who are infectious. The social realities of family structures, housing conditions, and much else will be anecdotally familiar to anyone responsible for the staff in a large department (for example, parents with children in school are more likely to catch colds). Much relevant work remains to be done in teasing apart the social, genetic, age-related, and other complications that are smoothed out in the usual mass action assumption.

In a country such as the United Kingdom, where childhood vaccination [HN16] remains voluntary, the practical questions have more to do with fundamental problems in the evolution of altruistic behavior than with the ecological dynamics so beautifully surveyed by Earn and colleagues. There may be risks--very, very tiny, but not zero--associated with vaccination. For example, the risk of meningitis/encephalitis associated with MMR (measles, mumps, and rubella) vaccination [HN17] is estimated to be around 1 in 1,000,000 (5). The risks of infection itself are, however, much greater: from around 1 in 200 to 1 in 5000 for complications due to meningitis/encephalitis after natural infection with measles, mumps, or rubella (5). So, your best strategy is for everyone else's child to be vaccinated, thus eradicating the risk of infection, but for your own child to remain unvaccinated. But we are now trapped in the Prisoner's Dilemma [HN18]: If too many parents "cheat" in this way, herd immunity will no longer protect their children. This is a real paradox, with no trick answer. I believe that the answer to such dilemmas, where individual and group interests ineluctably conflict, is a program of effectively compulsory immunization, backed by a clear and analytical understanding of the effects of population level or "herd" immunity, which are so admirably illustrated by the Earn study.

References

1. R. M. Anderson and R. M. May, Infectious Diseases of Humans: Dynamics and Control (Oxford Univ. Press, Oxford, 1991).
2. W. H. McNeill, Plagues and Peoples (Blackwell, Oxford, 1976).
3. D. J. D. Earn et al., Science 287, 667 (2000).
4. R. M. May, in Biomathematics, T. Hallam and S. A. Levin, Eds. (Springer-Verlag, Berlin, 1986), vol. 17, pp. 405-442.
5. U.K. Parliamentary Office of Science and Technology, Note 131 (December 1999). See also parliament.uk/post/home.htm.

---

The author is Chief Scientific Adviser to the U.K. government, Office of Science and Technology, 94-98 Albany House, London SW1H 9ST, UK. E-mail: robert.may@zoo.ox.ac.uk

HyperNotes
Related Resources on the World Wide Web

General Hypernotes

The WWW Virtual Library: Epidemiology is maintained by the Department of Epidemiology and Biostatistics, University of California, San Francisco. The WWW Virtual Library of Microbiology and Virology is available from the Microbiology Network.

The library of the Karolinska Institutet, Stockholm, provides links to biomedical Web resources. Diseases and disorders (with a section on the history of diseases) and epidemiology and biostatistics are among the subject areas covered.

The School of Public Health, Queensland University of Technology, Kelvin Grove, Australia, provides links to Internet public health resources.

SciCentral offers links to Internet resources and articles on infectious diseases and epidemiology.

All the Virology on the WWW is maintained by D. Sanders, Department of Microbiology and Immunology, Tulane University School of Medicine.

J. Swinton, Department of Zoology, University of Cambridge, offers a dictionary of epidemiology.

The British Medical Journal (BMJ) makes available Epidemiology for the Uninitiated by D. Coggon, G. Rose, and D. Barker.

Medical Microbiology, an online textbook edited by S. Baron, Department of Microbiology and Immunology, University of Texas Medical Branch at Galveston, includes chapters on epidemiology, viral epidemiology, and paramyxoviruses (which include the measles virus).

T. Terry, Department of Molecular and Cell Biology, University of Connecticut, provides lecture notes on epidemiology and public health for a microbiology course.

Lectures on epidemiology prepared for an Internet course titled "Epidemiology, the Internet, and public health" are made available by R. LaPorte, Department of Epidemiology, Graduate School of Public Health, University of Pittsburgh.

Mathematical Modeling: Analysis of Epidemics is a tutorial by D. Short, College of Sciences, San Diego State University.

The Wellcome Trust Centre for the Epidemiology of Infectious Diseases, Oxford, UK, provides a brochure with background information and descriptions of research projects, as well as links to epidemiology resources on the Web.

The Mathematical Biology Pages is a tutorial offered by the Department of Life Sciences, Brandeis University, Waltham, MA.

J. Matthiopoulos, Gatty Marine Laboratory, University of St. Andrews, UK, provides a collection of links to mathematical biology sites.

The 17 January 1997 issue of Science had an article by S. Levin, B. Grenfell, A. Hastings, and A. Perelson titled "Mathematical and computational challenges in population biology and ecosystems science."

The 5 February 1999 issue of Science had an review article by B. Levin, M. Lipsitch, and S. Bonhoeffer titled "Population biology, evolution, and infectious disease: Convergence and synthesis."

J. Koopman, Department of Epidemiology, School of Public Health, University of Michigan, makes available a paper presented at a meeting titled "Epidemiology and human interactions: Emerging metaphors, models, and methods" and a commentary titled "Individual causal models and population system models in epidemiology." Koopman provides an introduction to modeling epidemiological processes in course notes on compartmental model analysis of epidemiologic processes.

Numbered Hypernotes

1. Epidemic! The World of Infectious Diseases is an online exhibit presented by the American Museum of Natural History. K. Nicholson, Department of Microbiology and Immunology, University of Leicester, UK, presents an introduction to the epidemiology of infectious diseases. The Encyclopædia Britannica article on communicable diseases, available from Britannica.com, includes an introduction to epidemiology. R. Hurlbert, Washington State University, provides lecture notes for a microbiology course that includes presentations on microbial disease mechanisms and epidemiology. P. Pugl, Department of Mathematics, University of Hartford, CT, presents lecture notes on the history of epidemics and plagues for a course on epidemics and AIDS; an introduction to epidemiology is included. The 30 July 1999 issue of Morbidity and Mortality Weekly Report (MMWR), published by the U.S. Centers for Disease Control and Prevention (CDC), had an article titled "Achievements in public health, 1900-1999: Control of infectious diseases."
2. A presentation on the Paramyxoviridae family of viruses (which includes the measles virus), a student project for a course on humans and viruses, is made available by R. Siegel, Department of Microbiology and Immunology, Stanford University. Britannica.com provides Encyclopædia Britannica articles about measles and the measles vaccine. The Merck Manual of Diagnosis and Therapy has a section on measles. The New York State Department of Health offers a fact sheet on measles. The U.K. Public Health Laboratory Service provides an information page on measles. A chapter on measles is included in the fourth edition of the textbook Epidemiology and Prevention of Vaccine-Preventable Diseases, which is available on the Web (in Adobe Acrobat format) from the National Immunization Program of the CDC. The 29 December 1989 supplement to MMWR titled "Measles prevention: Recommendations of the Immunization Practices Advisory Committee" included background information on measles epidemiology and elimination efforts.
3. The Doctors without Borders Web site provides information about diseases such as measles in the developing world. Removing Obstacles to Healthy Development is a 1999 report on infectious diseases from the World Health Organization (WHO). WHO's Diseases and Vaccines Web site provides information about measles. The Pan-American Health Organization describes its programs in disease prevention and control in the Americas and provides country health profiles; the Division of Vaccines and Immunization offers information about measles. The 11 December 1998 issue of MMWR had an article titled "Progress toward global measles control and regional elimination, 1990-1997."
4. HIV InSight is a comprehensive Web resource on HIV/AIDS provided by the University of California, San Francisco. UNAIDS is a joint United Nations program on HIV/AIDS, which provides AIDS documentation by topic and by region. The World Bank offers an HIV/AIDS information page. The U.S. National Institute for Allergy and Infectious Diseases (NIAID) provides a collection of information resources on AIDS. C. Toebe, Department of Biology, City College of San Francisco, provides lecture notes for a course on the biology of HIV. The WHO Initiative on HIV/AIDS and Sexually Transmitted Infections Web page provides information resources and Internet links.
5. The Animal and Plant Health Inspection Service of the U.S. Department of Agriculture provides information about bovine spongiform encephalopathy (BSE). The Department of Microbiology and Immunology, University of Leicester, presents a tutorial on BSE and a collection of links to recent BSE news. The WHO Communicable Disease, Surveillance and Response division provides information about BSE and other transmissible spongiform encephalopathies. The Wellcome Trust Centre for the Epidemiology of Infectious Diseases provides a research information page on transmissible spongiform encephalopathies. The 22 January 2000 issue of New Scientist had an article about BSE titled "A lesser evil: Human BSE may kill thousands, but it could have been worse."
6. The Anatomy of an Epidemic, a student ThinkQuest project, offers presentations on tuberculosis and mad cow disease (BSE). The Center for Tuberculosis at Johns Hopkins University provides information on the disease including a presentation on its epidemiology and natural history. The WHO tuberculosis Web site provides a tuberculosis fact sheet. A student presentation on tuberculosis was prepared for a course on vaccine development offered by the Program in Biology, Brown University, Providence, RI. Information about tuberculosis is provided by the CDC's Division of Tuberculosis Elimination. NIAID provides information about tuberculosis.
7. K. Todar, Department of Bacteriology, University of Wisconsin, presents lecture notes on antibiotic resistance for a course on host-parasite interactions; also available are lecture notes on tuberculosis by J. Harms. M. Clarke, Department of Microbiology and Immunology, University of Western Ontario, provides lecture notes on drug resistance for a course on the biology of infection and immunity. The WHO report on infectious diseases includes a chapter on drug resistance. WHO's Communicable Disease Surveillance and Response division provides information about anti-infective drug resistance. NIAID presents information on antimicrobial resistance. The March 1998 issue of Scientific American had an article by S. Levy titled "The challenge of antibiotic resistance."
8. D. Earn is in the Department of Mathematics and Statistics, McMaster University, Hamilton, Ontario, Canada. P. Rohani and B. Grenfell are in the Parasite Population Dynamics Group, Zoology Department, University of Cambridge. B. Bolker is in the Department of Zoology, University of Florida.
9. Swinton's epidemiology dictionary defines microparasites.
10. J. Koopman presents lecture notes on transmission system analysis for a course on advanced infectious disease epidemiology.
11. The Society for Chaos Theory in Psychology and Life Sciences (SCPLS), an international forum for researchers interested in the application of nonlinear dynamics and complex systems theory to the life sciences, offers a tutorial on basic concepts in nonlinear dynamics and chaos. S. Otto, Department of Zoology, University of British Columbia, provides lecture notes for a course on biomathematics; a presentation titled "Analysing non-linear equations: Spread of disease" is included.
12. An introduction to the SEIR model of infectious diseases is provided by the Edinburgh Parallel Computing Centre as part of a presentation titled "The SEIR Demonstrator: Spatial modelling of epidemics on high performance computers."
13. The 29 October 1996 issue of the Proceedings of the National Academy of Sciences had an article by B. Bolker and B. Grenfell titled "Impact of vaccination on the spatial correlation and persistence of measles dynamics." The 29 October 1999 issue of Science had an article by P. Rohani, D. Earn, and B. Grenfell titled "Opposite patterns of synchrony in sympatric disease metapopulations."
14. Swinton's epidemiology dictionary defines basic reproductive ratio.
15. The SCPLS nonlinear dynamics tutorial includes an introduction to the bifurcation diagram. The Nonlinear Lab, a Web tutorial on chaotic systems by B. Fraser, includes a presentation on bifurcations.
16. Compton's Encyclopedia Online has an article about vaccines. The Vaccine Page provides access to news about vaccines and an annotated database of vaccine resources on the Internet. The About.com Guide to Nursing offers a feature titled "The impact of vaccines on human health"; a list of Web resources on vaccination is also provided. The 13 November 1999 issue of BMJ had an article by M. Liu titled "Vaccines in the 21st century." The Department of Microbiology and Immunology, University of Leicester, makes available lecture notes on viral vaccines for a microbiology course. A presentation on vaccines by R. Hunt, Department of Microbiology and Immunology, School of Medicine, University of South Carolina, is included in a collection of departmental virology lecture notes. The Diseases and Vaccines page from WHO's Department of Vaccines and Biologicals offers a history of vaccination and immunization profiles of countries, as well as a vaccine safety page. The CDC's National Vaccine Programs Office provides information on immunization, including sections on concepts, laws, and safety. The National Academy Press makes available on the Web the summary of a 1997 workshop titled "Risk communication and vaccination." The CDC's National Immunization Program offers a presentation titled "Vaccine safety: What you need to know," as well one titled "Why immunize?". The U.K. Health Education Authority Immunisation Programme provides fact sheets about topics such as MMR vaccinations.
17. The CDC's National Immunization Program provides information about the MMR vaccine. Adam.com provides information about MMR immunization. The U.K. Parliamentary Office of Science and Technology (POST) makes available a POSTnote briefing titled "Health concerns and the MMR vaccine" in Adobe Acrobat format (5).
18. The Prisoner's Dilemma is defined in the Stanford Encyclopedia of Philosophy. McGraw-Hill's Online Learning Center for L. Sdorow's Psychology offers an interactive presentation on the Prisoner's Dilemma. The Serendip Web site at Bryn Mawr College offers an information page about the Prisoner's Dilemma, as well as an interactive version of the game. R. McCain, Department of Economics, Drexel University, Philadelphia, offers a presentation on the Prisoner's Dilemma in his introduction to game theory.
19. R. May is Chief Scientific Adviser to the U.K. Office of Science and Technology; information about his research interests and recent publications is provided by the Mathematical Biology Group, Department of Zoology, University of Oxford.